Q:

Calculate the mass percentage of benzene (C₆H₆) and carbon tetrachloride (CCl₄) if 22 g of benzene is dissolved in 122 g of carbon tetrachloride.

A:

We know that:

Mass percentage of C₆H₆

and we also know that:

Mass percentage of CCl₄

Alternatively,

Mass percentage of CCl₄= (100 - 15.28)%

= 84.72%

Q:

Calculate the mole fraction of benzene in solution containing 30% by mass in carbon tetrachloride.

A:

Let the total mass of the solution be 100 g and the mass of benzene be 30 g.

∴ Mass of carbon tetrachloride = (100 - 30) g = 70 g

Molar mass of benzene (C₆H₆) = (6 × 12 + 6 × 1) g mol^{- 1}

= 78 g mol^{- 1}

∴ Number of moles of C₆H₆ =30/78 mol

= 0.3846 mol

Molar mass of carbon tetrachloride (CCl₄) = 1 × 12 + 4 × 35.5

= 154 g mol^{- 1}

∴ Number of moles of CCl₄ = 70/154 mol

= 0.4545 mol

Thus, the mole fraction of C₆H₆ is given as:

= 0.3846 / (0.3846 + 0.4545)

= 0.458

Q:

Calculate the molarity of each of the following solutions:

(a)30 g of Co(NO₃)₂. 6H₂O in 4.3 L of solution

(b)30 mL of 0.5 M H₂SO₄ diluted to 500 mL.

A:

Molarity is given by:

Molarity = moles of solute / Volume of solution in litre

(a) Molar mass of Co(NO₃)₂.6H₂O

= 59 + 2 (14 + 3 × 16) + 6 × 18 = 291 g mol^{- 1}

∴Moles of Co(NO₃)₂.6H₂O = 30 / 291 mol

= 0.103 mol

Therefore, molarity = 0.103 mol / 4.3 L

= 0.024 M

(b) Number of moles present in 1000 mL of 0.5 M H₂SO₄ = 0.5 mol

∴ Number of moles present in 30 mL of 0.5 M H₂SO₄ = (0.5 X 30 ) / 1000 mol

= 0.015 mol

Therefore, molarity = 0.015 mol / 0.5 L

= 0.03 M

Q:

Calculate the mass of urea (NH₂CONH₂) required in making 2.5 kg of 0.25 molal aqueous solution.

A:

Molar mass of urea (NH₂CONH₂) = 2 (1 × 14 + 2 × 1) + 1 × 12 + 1 × 16

= 60 g mol^{- 1}

0.25 molar aqueous solution of urea means:

1000 g of water contains 0.25 mol = (0.25 × 60) g of urea

= 15 g of urea

That is, (1000 + 15) g of solution contains 15 g of urea

Therefore, 2.5 kg (2500 g) of solution contains = (15 X 2500) / (1000+15) g

= 36.95 g

= 37 g of urea (approximately)

Hence, mass of urea required = 37 g

Q:

Calculate

(a) molality

(b) molarity and

(c) mole

fraction of KI if the density of 20% (mass/mass) aqueous KI is 1.202 g mL^-1.

A:

(a) Molar mass of KI = 39 + 127 = 166 g mol^{- 1}

20% (mass/mass) aqueous solution of KI means 20 g of KI is present in 100 g of solution.

That is,

20 g of KI is present in (100 - 20) g of water = 80 g of water

Therefore, molality of the solution = Moles of KI / Mass of water in kg

= 20/166 / 0.08 m

= 1.506 m

= 1.51 m (approximately)

(b) It is given that the density of the solution = 1.202 g mL^{- 1}

∴Volume of 100 g solution = Mass / Density

= 100g / 1.202g mL^-1

= 83.19 mL

= 83.19 × 10^{- 3}L

Therefore, molarity of the solution = 20/166 mol / 83.19 × 10^{- 3}L

= 1.45 M

(c) Moles of KI = 20/166 = 0.12 mol

Moles of water = 80/18 = 4.44 mol

Therefore, mole fraction of KI = Moles of KI / (Moles of KI + Moles of water)

= 0.12 / (0.12+4.44)

= 0.0263

Q:

H₂S, a toxic gas with rotten egg like smell, is used for the qualitative analysis. If the solubility of H₂S in water at STP is 0.195 m, calculate Henry's law constant.

A:

It is given that the solubility of H₂S in water at STP is 0.195 m, i.e., 0.195 mol of H₂S is dissolved in 1000 g of water.

Moles of water = 1000g / 18g mol^-1

= 55.56 mol

∴Mole fraction of H₂S, x = Moles of H₂S / Moles of H₂S + Moles of water

0.195 / (0.195 + 55.56)

= 0.0035

At STP, pressure (p) = 0.987 bar

According to Henry's law:

p= K_Hx

⇒ K_H = p / x

= 0.0987 / 0.0035 bar

= 282 bar

Q:

Henry's law constant for CO₂ in water is 1.67 x 10⁸Pa at 298 K. Calculate the quantity of CO₂in 500 mL of soda water when packed under 2.5 atm CO₂pressure at 298 K.

A:

It is given that:

K_H= 1.67 × 10⁸Pa

P_CO2= 2.5 atm = 2.5 × 1.01325 × 10⁵Pa

= 2.533125 × 10⁵Pa

According to Henry's law:

P_CO2= K_H^X

⇒ x = P_CO2 / K_H

= 2.533125 × 10⁵/ 1.67 × 10⁸

= 0.00152

We can write,

[Since, is negligible as compared to]

In 500 mL of soda water, the volume of water = 500 mL

[Neglecting the amount of soda present]

We can write:

500 mL of water = 500 g of water

=500 / 18 mole of water

= 27.78 mol of water

Now, n_CO2 / n_H2O = x

n_CO2 / 27.78 = 0.00152

n_CO2= 0.042 mol

Hence, quantity of CO₂in 500 mL of soda water = (0.042 × 44) g

= 1.848 g

Q:

The vapour pressure of pure liquids A and B are 450 and 700 mm Hg respectively, at 350 K. Find out the composition of the liquid mixture if total vapour pressure is 600 mm Hg. Also find the composition of the vapour phase.

A:

It is given that:

P_A^o = 450 mm of Hg

P_B^o= 700 mm of Hg

p_total= 600 mm of Hg

From Raoult's law, we have:

p_total= P_A+ P_B

Therefore, x_B = 1 - x_A

= 1 - 0.4

= 0.6

Now, P_A= P_A^ox_A

= 450 × 0.4

= 180 mm of Hg

and P_B= P_B^ox_B

= 700 × 0.6

= 420 mm of Hg

Now, in the vapour phase: Mole fraction of liquid A = P_A/ (P_A+ P_B )

=180 / (180+420)

= 180/600

= 0.30

And, mole fraction of liquid B = 1 - 0.30

= 0.70

Q:

Vapour pressure of pure water at 298 K is 23.8 mm Hg. 50 g of urea (NH₂CONH₂) is dissolved in 850 g of water. Calculate the vapour pressure of water for this solution and its relative lowering.

A:

It is given that vapour pressure of water,P_I^o = 23.8 mm of Hg

Weight of water taken, w₁= 850 g

Weight of urea taken, w₂= 50 g

Molecular weight of water, M₁= 18 g mol^{- 1}

Molecular weight of urea, M₂= 60 g mol^{- 1}

Now, we have to calculate vapour pressure of water in the solution. We take vapour pressure as p₁.

Now, from Raoult's law, we have:

Hence, the vapour pressure of water in the given solution is 23.4 mm of Hg and its relative lowering is 0.0173.

Q:

Boiling point of water at 750 mm Hg is 99.63°C. How much sucrose is to be added to 500 g of water such that it boils at 100°C.Molal elevation constant for water is 0.52 K kg mol^-1.

A:

Here, elevation of boiling point ΔT_b= (100 + 273) - (99.63 + 273)

= 0.37 K

Mass of water, w_l= 500 g

Molar mass of sucrose (C₁₂H₂₂O₁₁),

M₂= 11 × 12 + 22 × 1 + 11 × 16 = 342 g mol ^{- 1}

Molal elevation constant, K_b= 0.52 K kg mol^{- 1}

We know that:

= (0.37 x 342 x 500) / (0.52 x 1000)

= 121.67 g (approximately)

Hence, 121.67 g of sucrose is to be added.

Q:

Calculate the mass of ascorbic acid (Vitamin C, C₆H₈O₆) to be dissolved in 75 g of acetic acid to lower its melting point by 1.5°C. K_f= 3.9 K kg mol^-1.

A:

Mass of acetic acid, w₁= 75 g Molar mass of ascorbic acid (C₆H₈O₆),

M₂= 6 × 12 + 8 × 1 + 6 × 16

= 176 g mol^{- 1}

Lowering of melting point, ΔT_f= 1.5 K

We know that:

= (1.5 x 176 x 75) / (3.9 x 1000)

= 5.08 g (approx)

Hence, 5.08 g of ascorbic acid is needed to be dissolved.

Q:

Calculate the osmotic pressure in pascals exerted by a solution prepared by dissolving 1.0 g of polymer of molar mass 185,000 in 450 mL of water at 37°C.

A:

It is given that:

Volume of water, V= 450 mL = 0.45 L

Temperature, T = (37 + 273)K = 310 K

Number of moles of the polymer,

n = 1 / 185000 mol

We know that:

Osmotic pressure,

= 1/185000 mol X 1/0.45L X 8.314 X 10³ Pa L K^-1mol^-1 X 310K

= 30.98 Pa = 31 Pa (approximately)

Q:

Define the term solution. How many types of solutions are formed? Write briefly about each type with an example.

A:

A solution is a homogeneous mixtures of two or more than two substances on molecular level.The constitutent of the mixture present in a smaller amount is called the SOLUTE & the one present in larger amount is called the SOLVENT.For eg. Small amount of sugar(solute) dissolved in water(solvent).

SOLUTE + SOLVENT = SOLUTION

There are nine types of solutions formed.They are:

Sno	State of solute	State of solvent	Examples
1	GAS	GAS	Air
2	GAS	LIQUID	Oxygen in water
3	GAS	SOLID	Smole particles in air
4	LIQUID	GAS	Carbon dioxide dissolved in water
5	LIQUID	LIQUID	Alcohol in water
6	LIQUID	SOLID	Mercury in silver
7	SOLID	GAS	Adsorption of hydrogen over palladium
8	SOLID	LIQUID	Sugar in water
9	SOLID	SOLID	Carbon in Iron(steel)

Out of these nine types of solution , solid in liquid, liquid in liquid & gas in liquid are very common.When the components of the solution are mixed,the resulting solution may be in the solid, liquid or gaseous state.They are

(i) Gaseous solution:The solution in which the solvent is a gas is called a gaseous solution. In these solutions, the solute may be liquid, solid, or gas. For example, a mixture of oxygen and nitrogen gas is a gaseous solution.

(ii) Liquid solution:The solution in which the solvent is a liquid is known as a liquid solution. The solute in these solutions may be gas, liquid, or solid.

(iii) Solid solutions: The solution in which the solvent is a solid is known as a solid solution.The solute in these solutions may be a gas, liquid or solid. For example, a solution of copper in gold is a solid solution.

Q:

Suppose a solid solution is formed between two substances, one whose particles are very large and the other whose particles are very small. What kind of solid solution is this likely to be?

A:

The given solid solution is the example of interstitial solid solution.

For example: tungsten carbide, where tungsten atoms are arranged in a face centred cubic pattern with carbon atoms in octahedral holes.

Q:

Define the following terms:

(i) Mole fraction (ii) Molality (iii) Molarity (iv) Mass percentage.

A:

a) Mole Fraction - it is defined as the ratio of number of moles of one component to the total number of moles of solute & solvent present in the solution.

Mole fraction = number of moles of the component / total number of moles of all components

It is denoted by x.

Take it as binary solution, the number of the moles of solute will be n_Aand the number of moles of solvent will be n_B, then the mole fraction of the solute in the solution is given as:

In the same manner, the mole fraction of the solvent in the solution is given as:

b) Molality- it is the number of moles of the solute dissolved per 1000g of the solvent.

It is denoted by m

Molality = mole of solute / mass of solvent in kg

Unit for molality are moles/kg

c) Molarity- it is the number of moles of the solute dissolved per litre of the solution.

It is represented by M.

Molarity = moles of solute / volume of solute in litres

Unit for molarity is moles/litre

d) Mass Percentage- the mass percentage of a component in a given solution is the mass of the component per 100g of the solution.

Mass percentage of the component = (mass of the component in the solution/total mass of the solution) x100

Q:

Concentrated nitric acid used in laboratory work is 68% nitric acid by mass in aqueous solution. What should be the molarity of such a sample of the acid if the density of the solution is 1.504 g mL^-1?

A:

Concentrated nitric acid used in laboratory work is 68% nitric acid by mass in an aqueous solution. This means that 68 g of nitric acid is dissolved in 100 g of the solution.

Molar mass of nitric acid (HNO₃) = 1 × 1 + 1 × 14 + 3 × 16 = 63 g mol^{- 1}

Then, number of moles of HNO₃= 68 / 63 mol

= 1.08 mol

Also density = 1.504g/mL^-1 (given)

Therefore from the formula density = mass / volume, we get

Volume of solution = 1000/1.504 = 66.49 mL

Therefore molarity of nitric acid = (1.08/66.49) x 1000 = 16.24 M

Q:

A solution of glucose in water is labelled as 10% w/w, what would be the molality and mole fraction of each component in the solution? If the density of solution is 1.2 g mL^-1, then what shall be the molarity of the solution?

A:

10% w/w solution of glucose in water means that 10 g of glucose in present in 100 g of the solution i.e., 10 g of glucose is present in (100 - 10) g = 90 g of water.

Molar mass of glucose (C₆H₁₂O₆) = 6 × 12 + 12 × 1 + 6 × 16 = 180 g mol^{- 1}

Then, number of moles of glucose = 10 / 180 mol

= 0.056 mol

∴ Molality of solution = 0.056 mol / 0.09kg = 0.62 m

Number of moles of water = 90g / 18g mol^-1 = 5 mol

Mole fraction of glucose (x_g) = 0.056 / ( 0.056+5) = 0.011

And, mole fraction of water x_w= 1 - x_g

= 1 - 0.011 = 0.989

If the density of the solution is 1.2 g mL^{- 1}, then the volume of the 100 g solution can be given as:

= 100g / 1.2g mL^-1

= 83.33 mL

=83.33 x 10^-3L

∴ Molarity of the solution = 0.056 mol / 83.33 x 10^-3L

= 0.67 M

Q:

How many mL of 0.1 M HCl are required to react completely with 1 g mixture of Na₂CO₃ and NaHCO₃ containing equimolar amounts of both?

A:

let the amount of Na₂CO₃ be x

& that of NaHCO₃ be 1-x

Now moles of Na₂CO₃ = x / 106

& moles of NaHCO₃ = 1-x / 84

Now according to question , number of moles of Na₂Co₃ = number of moles of NaHCO₃

Therefore x / 106 = 1-x / 84

84x = 106-106x

84x +106x = 106

190x = 106

Or

x = 106 / 190 = 0.558

Therefore moles of Na₂Co₃ = 0.558 / 106 = 0.00526

&

moles of NaHCO₃ = 1 - 0.558 / 84 = 0.0053

Now Hcl reacts with Na₂Co₃ & NaHCO₃ as follows:

Na₂Co₃ + 2Hcl 2Nacl + H₂o + CO₂

NaHCO₃ + Hcl Nacl + H₂o + CO₂

From the above reactions, 1 mol of Na₂Co₃ will react with 2 mol of Hcl

Therefore 0.00526 mol of Na2Co₃ will react with 2 x 0.00526 mol of Hcl & similarly 0.00526 mol of NaHCO₃ will react with 0.00526 mol of Hcl

Total moles of Hcl required to react with mixture of of NaHCO₃ & Na₂Co₃

= 2 X 0.00526 + 0.00526 =0.01578 mol

Also according to question 0.1 mol of 0.1 M Hcl is present in 1000 ml

Or

0.01578 mol of 0.1 M Hcl is present in (1000/0.1) x 0.01578 = 157.8 ml

Hence, 158 mL of 0.1 M of HCl is required to react completely with 1 g mixture of Na₂CO₃ and NaHCO_3, containing equimolar amounts of both.

Q:

A solution is obtained by mixing 300 g of 25% solution and 400 g of 40% solution by mass. Calculate the mass percentage of the resulting solution.

A:

According to question,

300g of 25% solution contains solute = (300 x 25) / 100 = 75g

&

400g of 40% solution contains solute = (400 x 40) / 100 = 160g

Total solute = 75 + 160 = 235g

As given in the question, total solution =300 + 400 = 700g

Percentage of solute in the final solution = (235 x100) / 700 = 33.57%

Percentage of water in the final solution = 100 - 33.5 = 65.43%

Q:

An antifreeze solution is prepared from 222.6 g of ethylene glycol (C₂H₆O₂) and 200 g of water. Calculate the molality of the solution. If the density of the solution is 1.072 g mL^-1, then what shall be the molarity of the solution?

A:

Calculation of Molality :

Mass of ethylene glycol = 222.6 (Given)

Molar mass of ethylene glycol [C₂H₄(OH)₂]

= 2 X 12 + 6 x 1 + 2 x 16

= 62

Therefore moles of ethylene glycol

= 222.6g / 62 gmol^-1

= 3.59 mol

Mass of water = 200g (Given)

Therefore molality of the solution is = (moles of ethylene glycol / mass of water) x 1000

= (3.59 / 200) x 1000

= 17.95 m

Calculation of Molarity:

Moles of ethylene glycol = 3.59 mol (already calculated)

Total Mass of solution = 200 + 222.6

= 422.6g

Volume of solution = mass / density volume

= 422.6 / 1.072

= 394.22 ml

now molarity of the solution is = (moles of ethylene glycol / volume of solution) x 1000

= (3.59 / 394.22) x 1000

= 9.11 M

Q:

A sample of drinking water was found to be severely contaminated with chloroform (CHCl₃) supposed to be a carcinogen. The level of contamination was 15 ppm (by mass):

(i) express this in percent by mass

(ii) determine the molality of chloroform in the water sample.

A:

1) 15 ppm means : 15 parts per million(10⁶) of the solutions

So, Percent by mass = (mass of chloroform / total mass) x 100

= (15 / 10⁶) x 100

= 1.5 x 10^-3 %

2) Molality Mass of chloroform = 15 g

Molar mass of chloroform (CHCl₃) = 1 × 12 + 1 × 1 + 3 × 35.5

= 119.5 g mol^{- 1}

Moles of chloroform = 15 / 119.5 = 0.1255 mol

Mass of water = 10⁶

Therefore molality = (moles of chloroform / mass of water ) x 1000

= (0.1255 / 10⁶) x 1000

= 1.255 x 10^-4 m

Q:

What role does the molecular interaction play in a solution of alcohol and water?

A:

The lower members of alcohols are highly soluble in water but the solubility decreases with increase in the molecular weight. The solubility of lower alcohols in water is due to formation of hydrogen bonds(Hydrogen bonding) between alcohols & water molecules.

However, as the size of alcohol molecule increases, the alkyl groups becomes larger & prevents the formation of hydrogen bonds with water, & hence the solubility goes on decreasing with increase in the length of carbon chain.Also the interaction between the molecules of alcohol and water is weaker than alcohol−alcohol and water−water interactions. As a result, when alcohol and water are mixed, the intermolecular interactions become weaker and the molecules can easily escape. This increases the vapour pressure of the solution, which in turn lowers the boiling point of the resulting solution.

Q:

Why do gases always tend to be less soluble in liquids as the temperature is raised?

A:

The dissolution of a gas in a liquid is exothermic process.Therefore according to LeChatelier principle,with the increase in temperature,the equilibrium shifts in the backward direction.

Gas + Liquid → Solution + Heat

Therefore the solubility of gas in solution decreases with rise in temperature.

Q:

State Henry's law and mention some important applications?

A:

Henry’s law states that the mass of a gas dissolved per unit volume of the solvent at a given temperature is proportional to the pressure of the gas in equilibrium with the solution.

Or

It also states that the pressure of a gas over a solution in which the gas is dissolved is proportional to the mole fraction of the gas dissolved in the solution.

The important applications of Henry’s law are as follows:

1) In the production of carbonated beverages-in order to increase the solubility of CO₂ in cold drinks,beer etc., the bottle are sealed under high pressure. When the bottle is opened under normal atmospheric pressure, the pressure inside the bottle falls to atmospheric pressure & the excess CO₂ bubbles out of the bottle causing effervescence.

2) At high altitudes-the partial pressure of oxygen at high altitudes is less than the ground level. This results in low concentration of oxygen in the blood & tissues of the peoples.

3) In scuba diving- during scuba diving,when the diver breaths in compressed air from the supply tank, more nitrogen dissolves in the blood & other body fluids because the pressure at that depth is far greater than the surface atmospheric pressure.

Q:

The partial pressure of ethane over a solution containing 6.56 x 10^-3 g of ethane is 1 bar. If the solution contains 5.00 x 10^-2 g of ethane, then what shall be the partial pressure of the gas?

A:

According to Henry’s law

.m = k x p

Substituting the given values in the above equation.

We get

6.56 x10^-3 = k x 1

Or

k = 6.56 x10^-3

Now when m = 5 x 10^-2,

Then again substituting the given values in Henry’s law equation, we get

5 x 10^-2 = 6.56 x10^-3 x p

Or

p = 7.62 bar

Q:

What is meant by positive & negative deviations from raoult’s law & how is the sign of ΔmixH related to positive & negative deviations from Raoult’s law?

A:

Raoult’s law states that at a given temperature, the vapour pressure of a solution containing non volatile solute is directly proportional the mole fraction of the solvent.

Non ideal solutions shows positive & negative deviations from ideal behavoiur.

Non ideal solutions showing positive deviations from Raoult’s law- Consider a binary solution of two components A & B .If the A-B interaction in the solutions are weaker than A-A & B-B interactions in the two liquids forming the solution,then the escaping tendency of A & B types of molecules from the solution becomes more than from pure liquids.

As a result ,each component of solution has a partial vapour pressure greater than expected on the basis of Raoult’s law.This is called positive deviations from Raoult’s law,i.e P_A> P_A °x_A & P_B >P_B°x_B

Δ_mixH is positive because energy is required to break A-A & B-B attractive forces.Hence endothermic process.

Non ideal solutions showing Negative deviations from Raoult’s law- in such solutions,the A-B interactions are stronger than A-A & B-B interactions .Due to stronger A-B interactions ,the escaping tendency of A & B types of molecules from the solution becomes less than from pure liquids. Consequently, each component of the solution has a partial vapour pressure less than expected on the basis of Raoults law. This is called negative deviations form Raoults law,i.e P_A< P_A °x_A & P_B B°x_B

Δ_mixH is negative because energy is released due to increase in attractive forces. Hence exothermic process.

Q:

An aqueous solution of 2% non-volatile solute exerts a pressure of 1.004 bar at the normal boiling point of the solvent. What is the molar mass of the solute?

A:

Here,

Vapour pressure of the solution at normal boiling point (p₁) = 1.004 bar (Given)

Vapour pressure of pure water at normal boiling point (p₁⁰) = 1.013 bar

Mass of solute, (w₂) = 2 g

Mass of solvent (water), (w₁) = 100 - 2 = 98 g

Molar mass of solvent (water), (M₁) = 18 g mol^{- 1}

According to Raoult's law,

(p₁⁰ - p₁) / p₁⁰= (w₂x M₁) / (M₂x w₁ )

(1.013 - 1.004) / 1.013 = (2 x 18) / (M₂ x 98 )

0.009 / 1.013 = (2 x 18) / (M₂ x 98 )

M₂ = (2 x 18 x 1.013) / (0.009 x 98)

M₂ = 41.35 g mol^{- 1}

Hence, the molar mass of the solute is 41.35 g mol^{- 1}.

Q:

Heptane and octane form an ideal solution. At 373 K, the vapour pressures of the two liquid components are 105.2 kPa and 46.8 kPa respectively. What will be the vapour pressure of a mixture of 26.0 g of heptane and 35 g of octane?

A:

Vapour pressure of heptane p₁⁰ = 105.2 kPa

Vapour pressure of octane p_²⁰= 46.8 kPa

As we know that, Molar mass of heptane (C₇H₁₆) = 7 × 12 + 16 × 1 = 100 g mol ^{- 1}

∴ Number of moles of heptane = 26/100 mol = 0.26 mol

Molar mass of octane (C₈H₁₈) = 8 × 12 + 18 × 1 = 114 g mol^{- 1}

∴ Number of moles of octane = 35/114 mol = 0.31 mol

Mole fraction of heptane, x₁ = 0.26 / 0.26 + 0.31

= 0.456

And, mole fraction of octane, x₂ = 1 - 0.456 = 0.544

Now, partial pressure of heptane, p₁= x₂p₂⁰

= 0.456 × 105.2

= 47.97 kPa

Partial pressure of octane,p₂= x₂p₂⁰

= 0.544 × 46.8 = 25.46 kPa

Hence, vapour pressure of solution, p_total= p1 + p2

= 47.97 + 25.46

= 73.43 kPa

Q:

The vapour pressure of water is 12.3 kPa at 300 K. Calculate vapour pressure of 1 molal solution of a non-volatile solute in it.

A:

1 molal solution means 1 mol of the solute is present in 1000 g of the solvent (water).

Molar mass of water = 18 g mol^{- 1}

∴ Number of moles present in 1000 g of water = 1000/18

= 55.56 mol

Therefore, mole fraction of the solute in the solution is

x₂ = 1 / (1+55.56) = 0.0177.

It is given that,

Vapour pressure of water, p₁⁰= 12.3 kPa

Applying the relation, (P₁⁰- P₁) / P₁⁰= X₂

⇒ (12.3 - p₁) / 12.3 =0.0177

⇒ 12.3 - P₁ = 0.2177

⇒ p₁ = 12.0823

= 12.08 kPa (approximately)

Hence, the vapour pressure of the solution is 12.08 kPa.

Q:

Calculate the mass of a non-volatile solute (molar mass 40 g mol^-1) which should be dissolved in 114 g octane to reduce its vapour pressure to 80%.

A:

Let the vapour pressure of pure octane be p₁⁰.

Then, the vapour pressure of the octane after dissolving the non-volatile solute is 80/100 p₁⁰ = 0.8 p₁⁰.

Molar mass of solute, M₂ = 40 g mol ^{- 1}

Mass of octane, w₁ = 114 g

Molar mass of octane, (C₈H₁₈), M₁ = 8 × 12 + 18 × 1 = 114 g mol^{- 1}

Applying the relation,

(p₁⁰ - p₁) / p₁⁰= (w₂x M₁) / (M₂x w₁ )

⇒ (p₁⁰ - 0.8 p₁⁰) / p₁⁰= (w₂x 114) / (40 x 114 )

⇒ 0.2 p₁⁰ / p₁⁰= w₂/ 40

⇒ 0.2 = w₂/ 40

⇒ w₂ = 8 g

Hence, the required mass of the solute is 8 g.

Q:

A solution containing 30 g of non-volatile solute exactly in 90 g of water has a vapour pressure of 2.8 kPa at 298 K. Further, 18 g of water is then added to the solution and the new vapour pressure becomes 2.9 kPa at 298 K. Calculate:

1) Molar mass of the solute

2) Vapour pressure of water at 298 K.

A:

Let, the molar mass of the solute be M g mol ^{- 1}

Now, the no. of moles of solvent (water),n₁= 90g / 18g mol^-1

And, the no. of moles of solute,n₂= 30g / M mol^-1 = 30 / M mol

p₁= 2.8 kPa

Applying the relation:

(p₁⁰ - p₁) / p₁⁰= n₂/ (n₁+ n₂)

⇒ (p₁⁰ - 2.8) / p₁⁰= (30/M)/ {5+ (30/M)}

⇒ 1 - (2.8/p₁⁰) = (30/M) / {(5M+30)/M}

⇒ 1 - (2.8/p₁⁰) = 30 / (5M + 30)

⇒ 2.8/p₁⁰= 1 - 30 / (5M + 30)

⇒ 2.8/p₁⁰ = (5M + 30 - 30) / (5M + 30)

⇒ 2.8/p₁⁰= 5M / (5M+30)

⇒ p₁⁰ / 2.8 = (5M+30) / 5M ----------------(1)

After the addition of 18 g of water:

n₁ = (90+18g) / 18 = 6 mol

and the new vapour pressure is p₁ = 2.9 kPa (Given)

Again, applying the relation:

(p₁⁰ - p₁) / p₁⁰= n₂/ (n₁+ n₂)

⇒ (p₁⁰ - 2.9) / p₁⁰= (30/M)/ {6+ (30/M)}

⇒ 1 - (2.9/p₁⁰) = (30/M) / {(6M+30)/M}

⇒ 1 - (2.9/p₁⁰) = 30 / (6M + 30)

⇒ 2.9/p₁⁰= 1 - 30 / (6M + 30)

⇒ 2.9/p₁⁰ = (6M + 30 - 30) / (6M + 30)

⇒ 2.9/p₁⁰= 6M / (6M+30)

⇒ p₁⁰ / 2.9 = (6M+30) / 6M ----------------(2)

Dividing equation (1) by (2),we get:

2.9 / 2.8 = {(5M+30) / 5M} / {(6M+30) / 6M}

⇒ 2.9 x (6M+30 / 6) = (5M+30 / 5) x 2.8

⇒ 2.9 x (6M +30) x 5 = (5M+30) x 2.8 x 6

⇒ 87M + 435 = 84M + 504

⇒ 3M = 69

⇒ M = 23u

Therefore, the molar mass of the solute is 23 g mol - 1.

(ii) Putting the value of 'M' in equation (i), we get:

⇒ p₁⁰ / 2.8 = (5M+30) / 5M

⇒ p₁⁰ / 2.8 = (5x23+30) / 5x23

⇒ p₁⁰= (145 x 2.8) / 115

⇒ p₁⁰= 3.53

Hence, the vapour pressure of water at 298 K is 3.53 kPa.

Q:

A 5% solution (by mass) of cane sugar in water has freezing point of 271 K. Calculate the freezing point of 5% glucose in water if freezing point of pure water is 273.15 K.

A:

In case of cane sugar:

ΔT_f= (273.15 - 271) K = 2.15 K

Molar mass of sugar (C₁₂H₂₂O₁₁) = 12 × 12 + 22 × 1 + 11 × 16 = 342 g mol ^{- 1}

5% solution (by mass) of cane sugar in water means 5 g of cane sugar is present in (100 - 5)g = 95 g of water.

Now, number of moles of cane sugar =5/342 mol

= 0.0146 mol

Therefore, molality of the solution,m =0.0146mol / 0.095kg

= 0.1537 kg mol^{- 1}

Now applying the relation,

ΔT_f = K_f × m

⇒ K_f = ΔT_f / m

⇒ 2.15K / 0.1537 kg mol^-1

= 13.99 K kg mol^-1

Molar of glucose (C₆H₁₂O₆) = 6 × 12 + 12 × 1 + 6 × 16 = 180 g mol^{- 1}

5% glucose in water means 5 g of glucose is present in (100 - 5) g = 95 g of water.

∴ Number of moles of glucose = 5/180 mol

= 0.0278 mol

Therefore, molality of the solution, m =0.0278 mol / 0.095 kg

= 0.2926 mol kg^{- 1}

Applying the relation,

ΔTf = Kf × m

= 13.99 K kg mol^{- 1} × 0.2926 mol kg ^{- 1}

= 4.09 K (approximately)

Hence, the freezing point of 5% glucose solution is (273.15 - 4.09) K= 269.06 K.

Q:

Two elements A and B form compounds having formula AB₂ and AB₄. When dissolved in 20 g of benzene (C₆H₆), 1 g of AB₂ lowers the freezing point by 2.3 Kwhereas 1.0 g of AB₄ lowers it by 1.3 K. The molar depression constant for benzene is 5.1 Kkg mol^-1. Calculate atomic masses of A and B.

A:

As We know that:

M_B = (K_f x w_B x 1000) / (w_A X ΔT_f)

Now ΔT_f = 2.3 , w_B = 1.0 , w_A = 20, K_F = 5.1 (given)

PUTTING THE VALUES IN THE EQUATION

M_B = (5.1 x 1 x 1000) / (20 x 2.3) = 110.87 g/mol

Therefore M_AB2 = 110.9

For AB₄ compound

ΔTf = 1.3 , w_b = 1 ,w_a = 20

M_B = (5.1 X 1 X 1000) / (20 X 1.3) = 196 g/mol

Therefore M_AB = 196

Let x be the atomic mass of A & y be the atomic mass of B,

THEN M_AB2 = x + 2y = 110.9 ----------------------------------(1)

And M_AB = x + 4y = 196 ----------------------------------(2)

Subtracting 2 from 1 ,we get

2y = 196-110.9

y = 85.1 / 2

y = 42.6

Putting the value of y in 1 we get

x = 110.9 - 2 x 42.6

x = 25.7

Therefore atomic mass of A = 25.7 u Atomic mass of B = 42.6 u.

Q:

At 300 K, 36 g of glucose present in a litre of its solution has an osmotic pressure of 4.98 bar. If the osmotic pressure of the solution is 1.52 bars at the same temperature, what would be its concentration?

A:

Here we have given

π₁= 4.98

π₂ = 1.52

C₁ = 36/180

C₂ = ? (we have to find)

Now according to van’t hoff equation

Π = CRT

Putting the values in above equation,we get

4.98 = 36/180RT ------------------------1

1.52 = c₂RT ------------------------2

Now dividing equation 2 by 1 ,we get

(c₂ x 180) / 36 = 1.52 / 4.98

or

c₂ = 0.061

Therefore concentration of 2nd solution is 0.061 M

Q:

Suggest the most important type of intermolecular attractive interaction in the following pairs.

(i) n-hexane and n-octane

(ii) I₂ and CCl₄

(iii) NaClO₄ and water

(iv) methanol and acetone

(v) acetonitrile (CH₃CN) and acetone (C₃H₆O).

A:

1) both of them has van der waals interactions

2) both of them has van der waals interactions

3) both of them has ion dipole interactions

4) both of them has hydrogen bonding

5) both of them has dipole-dipole interactions

Q:

Based on solute-solvent interactions, arrange the following in order of increasing solubility in n-octane and explain. Cyclohexane, KCl, CH₃OH, CH₃CN.

A:

n-octane is an organic solvent (liquid)

Out of given examples cyclohexane is strongest organic solvent, so according to like dissolves like, cyclohexane is most likely to be dissolved as a solute in n octane. After cyclohexane, CH₃CN

Will be dissolved completely as a solute in n octane, then CH₃OH & the least soluble will be Kcl. Also since n-octane & cyclohexane, both of them belongs to alkane category, their solubility will be maximum.

Therefore the order of increasing solubility in n octane is as follows:

Kcl < CH₃OH < CH₃CN < Cyclohexane

Q:

Amongst the following compounds, identify which are insoluble, partially soluble and highly soluble in water?

(i) phenol (ii) toluene (iii) formic acid (iv) ethylene glycol (v) chloroform (vi) pentanol.

A:

(i) Phenol (C₆H₅OH) has the polar group -OH and non-polar group -C₆H₅. Thus, phenol is partially soluble in water.

(ii) Toluene (C₆H₅-CH₃) has no polar groups. Thus, toluene is insoluble in water.

(iii) Formic acid (HCOOH) has the polar group -OH and can form H-bond with water. Thus, formic acid is highly soluble in water.

(iv) Ethylene glycol has polar -OH group and can form H-bond. Thus, it is highly soluble in water.

(v) Chloroform is insoluble in water.

(vi) Pentanol (C₅H₁₁OH) has polar -OH group, but it also contains a very bulky non-polar -C₅H₁₁ group. Thus, pentanol is partially soluble in water.

Q:

If the density of some lake water is 1.25 g mL^-1 and contains 92 g of Na⁺ ions per kg of water, calculate the molality of Na⁺ ions in the lake.

A:

We know molality = moles of solute / mass of solvent in kg

Now mass of Na⁺ ions = 92 g (given)

Moles of Na⁺ ions = 92 / 23 = 4

And mass of water = 1kg

Therefore molality = 4/1 = 4m

Q:

If the solubility product of CuS is 6 x 10^-16, calculate the maximum molarity of CuS in aqueous solution.

A:

Solubility product of CuS, K_sp of CuS = 6 x 10^-16

If s is the solubility,

then CuS = cu²⁺ + S^2-

Therefore K_SP = { cu²⁺}{ S^2-}

Or

K_SP = s x s

Or

s = √ K_SP = √6 x 10^-16

= 2.45 x 10^-8 M

Q:

Calculate the mass percentage of aspirin (C₉H₈O₄) in acetonitrile (CH₃CN) when 6.5 g of C₉H₈O₄ is dissolved in 450 g of acetonitrile (CH₃CN).

A:

Mass of aspirin (C₉H₈O₄) = 6.5g (given)

Mass of acetonitrile (CH₃CN) = 450g (given)

Now total mass of the solution = 6.5 + 450 = 456.5g

Therefore mass percentage of aspirin (C₉H₈O₄) = (6.5 / 456.5 ) x 100

= 1.42 %

Q:

Nalorphene (C₁₉H₂₁NO₃), similar to morphine, is used to combat withdrawal symptoms in narcotic users. Dose of nalorphene generally given is 1.5 mg. Calculate the mass of 1.5 x 10^-3m aqueous solution required for the above dose.

A:

Molecular mass of nalorphene (C₁₉H₂₁NO₃),

= 19 x 12 + 21 x 1 + 1 x 14 + 3 x 16 = 311 g/mol

Moles of nalorphene (C₁₉H₂₁NO₃) = 1.5 x 10^-3 x 311 = 4.67 x 10^-6 mol

Now molality = (moles of solute / mass of solvent in g) x 1000

Putting the values in above equation,we get

1.5 x 10^-3 = (4.67 x 10^-6 / mass of water) x 1000

Or

Mass of water = (4.67 x 10^-6 x 1000) / 1.5 x 10^-3

Therefore mass of water = 3.1g

Q:

Calculate the amount of benzoic acid (C₆H₅COOH) required for preparing 250 mL of 0.15 M solution in methanol.

A:

We know Molarity = moles of the solute / volume of solution

Putting the given values in above equation,we get

0.15 = (mole of benzoic acid / 250) x 1000

Or

moles of benzoic acid = (0.15 x 250) / 1000

= 0.0375 mol of benzoic acid

Also molecular mass of benzoic acid (C₆H₅COOH)

= 7 × 12 + 6 × 1 + 2 × 16

= 122 g/mol

Therefore amount of benzoic acid = 0.0375 x 122 = 4.575 g

Q:

The depression in freezing point of water observed for the same amount of acetic acid, trichloroacetic acid and trifluoroacetic acid increases in the order given above. Explain briefly.

A:

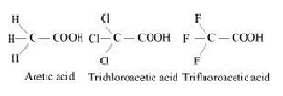
The above trend in the depression in the freezing point of water for the same amount of given compounds can be explained on the basis of degree of ionization, which depends upon the strength of the acid.Trifluroacetic acid is more acidic than trichloroacetic acid which is further more acidic than acetic acid.

Therefore the degree of ionization of these acids will decrease in the following order:

Trifluoroactic acid > trichloroacetic acid > acetic acid

Now greater the degree of ionization ,greater will be the depression of freezing point.

Q:

Calculate the depression in the freezing point of water when 10 g of CH₃CH₂CHClCOOH is added to 250 g of water. K_a = 1.4 x 10^-3, K_f = 1.86 K kg mol^-1.

A:

Molar mass of CH₃CH₂CHClCOOH

15 + 14 + 13 + 35.5 + 12 + 16 + 16 + 1

= 122.5 g/mol

∴ Moles of CH₃CH₂CHClCOOH = 10g / 122.5 g/mol

= 0.0816 mol

Therefore molality of the solution

= (0.0816 x 1000) / 250

= 0.3265 mol kg^-1

Now if a is the degree of dissociation of CH₃CH₂CHClCOOH,

So, K_a = (Cα x Cα) / (C (1-α))

K_a= Cα²/ (1-α)

Since α is very small with respect to 1, 1 - α = 1

K_a= Cα²

α = √K_α / C

Putting the values ,We get

α = √1.4 x 10^-3/ 0.3265

= 0.0655

Now at equilibrium,the van’t hoff factor i = 1-α +α +α/1

= 1 + 0.0655

= 1.0655

Hence, the depression in the freezing point of water is given as:

Therefore ΔT_f = i K_f m v

= 1.065 v x 1.86 x 0.3265

= 0.647°

Q:

19.5 g of CH₂FCOOH is dissolved in 500 g of water. The depression in the freezing point of water observed is 1.0°C. Calculate the van't Hoff factor and dissociation constant of fluoroacetic acid.

A:

Molecular mass of CH₂FCOOH

14 + 19 + 12 + 16 + 16 + 1 = 78 g/mol

Now, Moles of CH₂FCOOH = 19.5 / 78

= 0.25

Taking the volume of the solution as 500 mL, we have the concentration:

C = (0.25 / 500) X 1000

Therefore Molality = 0.50m

So now putting the value in the formula :

ΔT_f = K_f x m

=1.86 x 0.50 = 0.93K

Van’t hoff factor = observed freezing point depression / calculated freezing point depression

= 1 / 0.93 = 1.0753

Let α be the degree of dissociation of CH₂FCOOH

Now total number of moles = m(1-a) + ma +ma = m(1+a)

Or

i = α(1+α) / α = 1 +α = 1.0753

Therefore α = 1.0753- 1

= 0.0753

Now the Value of K_a is given as:

K_a= [CH₂FCOO^-][H⁺] / CH₂FCOOH

= (Cα x Cα) / (C (1-α))

= Cα²/ (1-α)

K_a = 0.5 X (0.0753)² / (1-0.0753)

= 0.5 X 0.00567 / 0.09247

= 0.00307 (approx.)

= 3 X 10^-3

Q:

Vapour pressure of water at 293 Kis 17.535 mm Hg. Calculate the vapour pressure of water at 293 Kwhen 25 g of glucose is dissolved in 450 g of water.

A:

Vapour pressure of water, p₁° = 17.535 mm of Hg

Mass of glucose, w₂ = 25 g

Mass of water, w₁ = 450 g

We know that,

Molar mass of glucose (C₆H₁₂O₆),

M₂= 6 × 12 + 12 × 1 + 6 × 16 = 180 g mol ^{- 1}

Molar mass of water, M₁ = 18 g mol ^{- 1}

Then, number of moles of glucose, n₁ = 25/180 = 0.139 mol

And, number of moles of water, n₂ =450/18 = 25 mol

Now, we know that,

(p₁° - p°) / p₁° = n1 / n₂ + n₁

⇒ 17.535 - p° / 17.535 = 0.139 / (0.139+25)

⇒ 17.535 - p₁ = 0.097

⇒ p₁ = 17.44 mm of Hg

Hence, the vapour pressure of water is 17.44 mm of Hg.

Q:

Henry's law constant for the molality of methane in benzene at 298 Kis 4.27 x 10⁵ mm Hg. Calculate the solubility of methane in benzene at 298 Kunder 760 mm Hg.

A:

As we know that

p = k x c

We have given p = 760 mm, k = 4.27 x 105

Putting the given values in equation

760 = 4.27 x 10⁵x c

Or

c = 760 / 4.27x 10⁵

c = 178 x 10^-5

Q:

100 g of liquid A (molar mass 140 g mol^-1) was dissolved in 1000 g of liquid B (molar mass 180 g mol^-1). The vapour pressure of pure liquid B was found to be 500 torr. Calculate the vapour pressure of pure liquid A and its vapour pressure in the solution if the total vapour pressure of the solution is 475 Torr.

A:

Number of Moles of Liquid A, n_A = 100 / 140 = 0.714

Number of Moles of Liquid B, n_B= 1000 / 180 = 5.556

Then Mole fraction of A = n_A / n_A + n_B = 0.714 / 0.714 + 5.556

= 0.114

Now Mole of fraction of B = 1 - 0.114 = 0.886

Now p_total = p_A + p_B

Or p_total = p°_AX_A + p°_Bx_B

OR

475 = p°_A X 0.114 + 500 X 0.886

OR

p°_A = 280.7 torr

Therefore vapour pressure of pure A = 280.7 torr

Vapour pressure of A in solution = 280.7 x 0.114

= 32 torr

Now

p_A= p°_AX_A

Or

p°_A= p_A/ X_A

⇒ 32 / 0.114

= 280.7 torr

Hence, the vapour pressure of pure liquid A is 280.7 torr.

Q:

Vapour pressure of pure acetone and chloroform at 328 K are 741.8 mm Hg and 632.8 mm Hg respectively. Assuming that they form ideal solution over the entire range of composition, plot p_total' p_chloroform' and p_acetoneas a function of x_acetone. The experimental data observed for different compositions of mixture is.

100 ×x_acetone	0	11.8	23.4	36.0	50.8	58.2	64.5	72.1
p_acetone /mm Hg	0	54.9	110.1	202.4	322.7	405.9	454.1	521.1
p_chloroform/mm Hg	632.8	548.1	469.4	359.7	257.7	193.6	161.2	120.7

A:

From the question, we have the following data

100 ×x_acetone	0	11.8	23.4	36.0	50.8	58.2	64.5	72.1
p_acetone /mm Hg	0	54.9	110.1	202.4	322.7	405.9	454.1	521.1
p_chloroform/mm Hg	632.8	548.1	469.4	359.7	257.7	193.6	161.2	120.7
p_total(mm Hg)	632.8	603.0	579.5	562.1	580.4	599.5	615.3	641.8

It can be observed from the graph that the plot for the p_totalof the solution curves downwards. Therefore, the solution shows negative deviation from the ideal behaviour.

Q:

Benzene and toluene form ideal solution over the entire range of composition. The vapour pressure of pure benzene and toluene at 300 K are 50.71 mm Hg and 32.06 mm Hg respectively. Calculate the mole fraction of benzene in vapour phase if 80 g of benzene is mixed with 100 g of toluene.

A:

Molar mass of benzene(C₆H₆) = 6 X 12 + 6 X 1 = 78 g/mol

Molar mass of toluene = 7 x 12 + 8 x 1 = 92 g/mol

Now no of moles in 80g of benezen = 80 / 78 = 1.026 mol

No of moles in 100g of toluene = 100 / 92 = 1.087 mol

∴Mole fraction of benzene x_b = 1.026 / 1.026 + 1.087 = 0.486

And Mole fraction of toluene,x_t = 1 - 0.486 = 0.514

We have given that

Vapor pressure of pure benzene p_b° = 50.71 mm Hg

And, vapour pressure of pure toluene, p_t° = 32.06 mm Hg

Therefore partial Vapor pressure of benzene, p_b = p_b X x_b

= 50.71 x 0.486

= 24.65 mm Hg

And partial Vapor pressure of toluene, p_t = p_t X x_t

P_t = p°_t X x_t = 32.06 x 0.514

= 16.48

Total vapour pressure = 24.65 + 16.48 = 41.13 mm Hg

Mole fraction of benzene in vapour phase = 24.65 / 41.13 = 0.60

Q:

The air is a mixture of a number of gases. The major components are oxygen and nitrogen with approximate proportion of 20% is to 79% by volume at 298 K. The water is in equilibrium with air at a pressure of 10 atm. At 298 Kif the Henry's law constants for oxygen and nitrogen are 3.30 x 10⁷ mm and 6.51 x 10⁷mm respectively, calculate the composition of these gases in water.

A:

Percentage of oxygen (O₂) in air = 20 %

Percentage of nitrogen (N₂) in air = 79%

Also, it is given that water is in equilibrium with air at a total pressure of 10 atm, that is, (10 × 760) mm Hg = 7600 mm Hg

Therefore, Partial pressure of oxygen, p_{o2 =}20/100 *7600

=1520 mm Hg

Partial pressure of nitrogen,p_N2= 79/100 *7600

= 6004 mmHg

Now, according to Henry's law:

p = K_H.x

For oxygen:

p_o2= K_H. x_O2

⇒x_O2= p_o2 / K_H

= 1520 / 3.30 X 10⁷

= 4.61 10^-5

For nitrogen:

p_N2 = K_H.x_N2

⇒x_N2= p_N2 / K_H

= 6004 / 6.51 x 10⁷

= 9.22 x 10^-5

Hence, the mole fractions of oxygen and nitrogen in water are 4.61 ×10 ^{- 5}and 9.22 × 10 ^{- 5}respectively.

Q:

Determine the amount of CaCl2 (i = 2.47) dissolved in 2.5 litre of water such that its osmotic pressure is 0.75 atm at 27°C.

A:

We know that

π = i n/V RT

⇒π = i w/MV iRT

⇒ w = πMV / iRT .......................(1)

Now we have given below values:

π = 0.75 atm

V = 2.5L

i = 2.47

T = (27+273) K = 300K

Here,

R = 0.0821L atm k^-1 mol^-1

M = 1x40 + 2x35.5

= 111 g/mol

Now putting the value in equation 1:

w = 0.75x111x2.5 / 2.47x0.0821x300

=3.42g

Hence, the required amount of CaCl₂ is 3.42 g.

Q:

Determine the osmotic pressure of a solution prepared by dissolving 25 mg of K₂SO₄ in 2 liter of water at 25° C, assuming that it is completely dissociated.

A:

If K₂SO₄ is completely dissociated then these ions are produced

Then K₂SO₄ = 2K⁺ + SO₄^2-

So total number of ions produced = 3

Therefore I =3

Now molecular mass of K₂SO₄ = 2 x 39 + 1 x 32 + 4 x 16 = 174 g/mol

Now π = I Crt

= I W_B X RT / M_B X V

= 3 X 25 X 10^-3 X 0.082 X 298 / 174 X 2

= 5.27 X 10^-3 atm.

Chapter 2 : Solutions

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 1 ( Page No. : 55 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Exercise 2 ( Page No. : 62 )

Students Also Read

Important links

Quick Links

Resources

Support